Have you ever found yourself in a situation where you need to express a part of a whole as a ratio out of 100? Perhaps you’re trying to calculate a discount on a product, figure out the proportion of students who passed a test, or determine the percentage of your salary that goes towards rent. In all these scenarios, understanding percentages becomes crucial. Today, we’ll delve into one such calculation – finding out what percentage 15 represents out of 60.
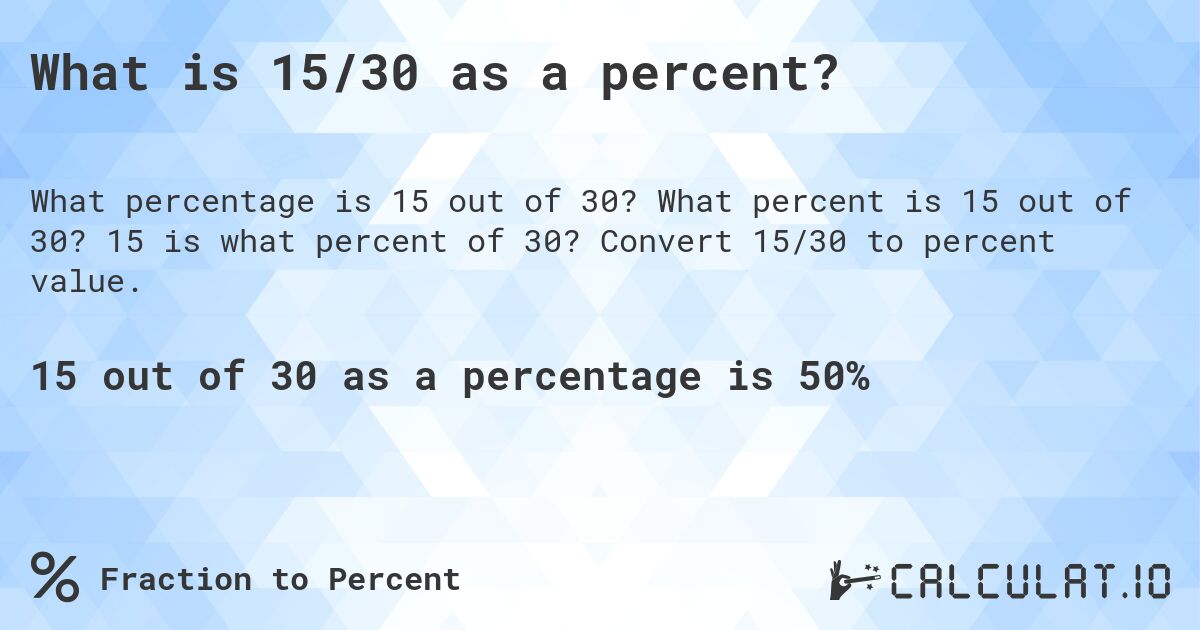
Image: calculat.io
At first glance, this might seem like a simple mathematical question. However, it’s worth exploring the context and the different ways you can approach this problem. Understanding the methodology behind calculating percentages can be incredibly valuable in a wide array of practical situations, making it a useful skill to master.
The Fundamentals of Percentages
The word “percentage” itself is derived from the Latin “per centum”, meaning “out of one hundred.” Essentially, a percentage represents a fraction out of 100 – a way of expressing a part relative to a whole. In our case, 15 is a part of the whole, which is 60. Our goal is to determine what portion of 100 this part represents.
Understanding Ratios
Before diving into the calculation, it’s important to understand ratios. A ratio is a way of comparing two quantities. In the context of the problem at hand, the ratio is 15:60, meaning that for every 60 units of the whole, there are 15 units of the part. This foundation helps us understand the relationship between the part and the whole.
Methods to Calculate the Percentage
There are a few different ways to calculate the percentage represented by 15 out of 60. Here are two commonly used methods:
Image: www.storyofmathematics.com
Method 1: Fraction and Conversion
1. **Form a fraction:** Represent the part (15) as the numerator and the whole (60) as the denominator. This gives us the fraction 15/60.
2. **Simplify the fraction:** Both 15 and 60 are divisible by 15. Dividing both numerator and denominator by 15, we get 1/4.
3. **Convert to percentage:** Multiply the simplified fraction (1/4) by 100%. (1/4) * 100% = 25%.
Method 2: Direct Calculation
1. **Divide the part by the whole:** Divide 15 by 60, which gives us 0.25.
2. **Multiply by 100:** Multiply the result by 100% to express it as a percentage. 0.25 * 100% = 25%.
Real-World Applications
This simple calculation, finding 15 as a percentage of 60, has numerous real-world applications. Here are some examples:
- Retail Sales: If a store offers a discount of 15% on a product originally priced at $60, the discount amount would be calculated as 25% of $60, resulting in a discount of $15.
- Academic Performance: If 15 students out of a class of 60 students pass an exam, the passing rate would be 25%.
- Financial Planning: If you allocate $15 out of a $60 budget for entertainment, you are dedicating 25% of your budget to entertainment.
- Survey Results: If 15 people out of 60 surveyed said they prefer a particular brand of coffee, this represents 25% of the respondents.
Beyond the Basics: Proportional Reasoning
Understanding percentages extends beyond basic calculations. It plays a crucial role in proportional reasoning, which is essential in various fields.
Proportional Reasoning in the Workplace
In the workplace, managers use proportional reasoning to allocate resources, plan budgets, and assess performance. For example, an HR manager might allocate a certain percentage of the company’s budget towards employee training based on the company’s overall goals and projected growth. Project managers use percentages to track progress and allocate resources based on project phases. Sales professionals often use percentages to track their performance, analyze sales trends, and forecast future revenue.
Proportional Reasoning in Science
Scientists use percentages in various experiments and analyses. For example, chemists use percentages to express the concentration of solutions. Physicists use percentages to calculate the efficiency of energy conversions. Biologists use percentages to analyze population growth rates and quantify the impact of environmental changes on species diversity.
Proportional Reasoning in Everyday Life
Even in everyday life, we use proportional reasoning frequently, often without realizing it. When cooking, we might adjust the ingredients based on the number of servings we need to prepare. When shopping, we might compare prices of different products to find the best deal, using percentages to help us determine the value for money. Even when tipping at a restaurant, we’re essentially using a percentage to express our appreciation for the service.
15 As A Percentage Of 60
Conclusion
Understanding the concept of percentages and the ability to calculate them accurately is a valuable skill. This seemingly simple mathematical concept has profound implications in various aspects of our lives. From making informed decisions about purchases to analyzing complex scientific data, percentages are an integral part of our daily lives. Next time you encounter a situation where you need to determine a part relative to a whole, remember the power of percentage calculations. You might be surprised by the insights and understanding they can offer.





