Have you ever wondered how to express a whole number like 13 as a fraction? It might seem like a simple question, but diving into the concept reveals a world of possibilities and intriguing insights into the language of mathematics. This seemingly straightforward inquiry delves into the heart of fractions, their diverse forms, and their application in various aspects of our daily lives. Understanding how to represent whole numbers as fractions opens doors to a deeper understanding of mathematical concepts and their relevance to real-world problems.
Image: www.slideshare.net
Fractions, in essence, are a way to represent parts of a whole. They consist of two numbers, a numerator and a denominator, separated by a horizontal line. The numerator tells us how many parts we have, while the denominator tells us how many equal parts the whole is divided into. For instance, 1/2 represents one out of two equal parts. But what happens when we want to express a whole number like 13 as a fraction? This is where the magic of equivalent fractions comes into play.
The Essence of Equivalent Fractions
The core principle behind representing a whole number as a fraction lies in the concept of equivalent fractions. Two fractions are considered equivalent if they represent the same quantity, even though they may look different. The key to generating equivalent fractions is to multiply both the numerator and the denominator by the same non-zero number.
Let’s illustrate this with an example. Consider the fraction 1/2. If we multiply both the numerator and the denominator by 2, we get (1 x 2) / (2 x 2) = 2/4. Even though the fractions 1/2 and 2/4 have different numerators and denominators, they represent the same value. This is because both fractions represent half of a whole.
Representing 13 as a Fraction
Now, let’s get back to our initial question: how do we represent 13 as a fraction? Since 13 is a whole number, we can simply write it as 13/1. This fraction implies that we have 13 parts out of a total of 1 part, essentially representing the complete whole. However, this is not the only way to represent 13 as a fraction. We can create infinitely many equivalent fractions by multiplying both the numerator and the denominator by the same non-zero number.
For instance, we can multiply both the numerator and the denominator of 13/1 by 2 to get (13 x 2) / (1 x 2) = 26/2. Likewise, we can multiply by 3 to get (13 x 3)/(1 x 3) = 39/3, and so on. We can generate an endless number of equivalent fractions, all representing the same whole number, 13. The choice of which fraction to use depends on the context of the problem or the desired level of precision.
Applications of Representing Whole Numbers as Fractions
The ability to express whole numbers as fractions opens up a world of possibilities in mathematics and its applications. It allows us to perform operations like addition, subtraction, multiplication, and division involving both fractions and whole numbers.
For example, suppose we need to add 13 to 1/4. We can first express 13 as the fraction 13/1. Now, we can simply add the numerators while keeping the denominator the same: (13 x 4) / (1 x 4) + 1/4 = (52 + 1) / 4 = 53/4.
This ability to work with whole numbers and fractions seamlessly is crucial in various fields, including:
- Cooking and Baking: Recipes often require fractions to accurately measure ingredients. The ability to represent whole numbers as fractions allows us to convert units easily and follow recipes precisely.
- Engineering and Architecture: Engineers and architects rely on fractions for precise measurements and calculations. Converting whole numbers to fractions ensures accurate and consistent designs.
- Finance and Accounting: Financial calculations often involve fractions to represent percentages, interest rates, and stock prices. Understanding how to use fractions in these contexts is crucial for accurate financial analysis.
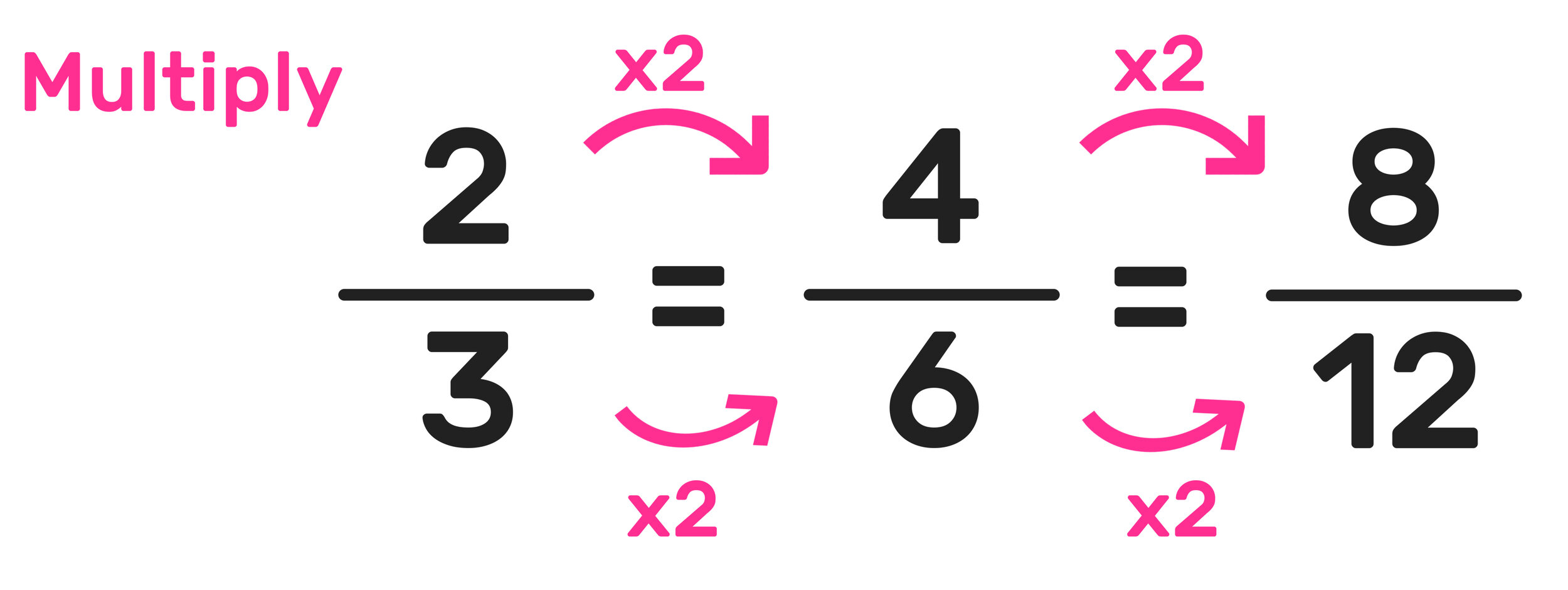
Image: www.elevate.in
The Importance of Understanding Equivalent Fractions
The concept of equivalent fractions is fundamental to understanding the relationship between whole numbers and fractions. It allows us to simplify complex fractions, find common denominators for addition and subtraction, and perform various mathematical operations with ease.
Equivalent fractions also play a vital role in understanding the concept of ratios and proportions. A ratio compares two quantities, while a proportion states that two ratios are equal. The ability to manipulate equivalent fractions is essential for solving problems involving ratios and proportions.
Beyond the Basics: Exploring the Depth of Fractions
While representing whole numbers as fractions might seem like a simple concept, it opens the door to a deeper understanding of the rich and multifaceted world of fractions. We can explore different types of fractions, including:
- Proper Fractions: Fractions where the numerator is smaller than the denominator, for example, 1/2, 2/3, and 3/4.
- Improper Fractions: Fractions where the numerator is greater than or equal to the denominator, for example, 5/3, 7/4, and 8/5.
- Mixed Numbers: A combination of a whole number and a proper fraction, for example, 2 1/2, 3 1/4, and 4 3/5.
Each type of fraction has unique characteristics and applications. Understanding these different types broadens our understanding of fractions and their role in various mathematical and real-world scenarios.
Whats 13 As A Fraction
Unlocking the Secrets of Fractions: A Journey of Discovery
Exploring the world of fractions is an ongoing journey of discovery, driven by curiosity and a passion for understanding the intricacies of mathematics. As we delve deeper into the concept, we uncover new insights, connections, and applications that enrich our mathematical understanding and broaden our perspectives.
From the simple act of representing a whole number as a fraction to mastering complex operations involving different types of fractions, our journey into the land of fractions is an enriching and rewarding experience. It opens our minds to the beauty and elegance of mathematics and its vast applications in shaping our world.





