Imagine a world where you’re tasked with dividing a delectable cake into three equal slices. Now imagine that this cake has 32 delicious bites! Suddenly, you’re confronted with a fascinating mathematical puzzle – how do you divide 32 bites into three equal portions? This is where the mathematical concept of division comes into play, and specifically, the question of 32 divided by 3. While it might seem like a simple arithmetic operation at first glance, delving deeper reveals a world of mathematical beauty, practicality, and insights that extend far beyond mere calculations.
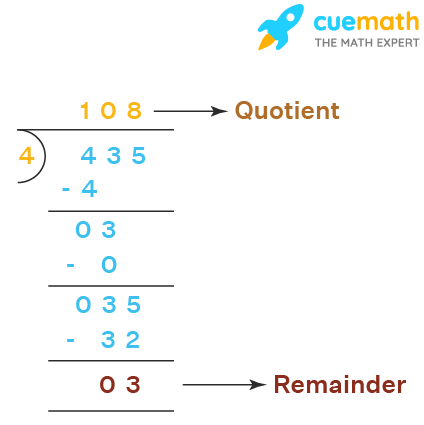
Image: ar.inspiredpencil.com
In this exploration, we embark on a journey through the fascinating world of 32 divided by 3, unraveling its intricacies and unearthing its significance in various aspects of our lives. From the basic principles of division to its applications in everyday scenarios, this article aims to empower you with a deeper understanding of this fundamental mathematical concept.
Understanding the Basics: Unveiling Division
At its core, division is the process of splitting a whole into equal parts. It’s a key operation in mathematics, serving as the foundation for various higher-level concepts. When we divide 32 by 3, we are essentially seeking to discover how many times 3 fits into 32. Just like our cake example, we aim to divide the 32 bites into three equal groups, and the result of this division will tell us how many bites would be in each of these groups.
The Result of 32 Divided by 3
Performing the actual calculation, we find that 32 divided by 3 yields an answer of 10 with a remainder of 2. What does this mean? It implies that 3 goes into 32 a total of 10 times, and we are left with 2 additional bites that cannot be equally divided into the three groups. This remainder is a crucial part of the result, as it signifies that the division is not perfectly clean, and there will always be 2 bites “left over.”
Beyond the Calculation: Real-World Applications of 32 Divided by 3
This seemingly simple calculation has a surprising range of applications in our daily lives. Beyond dividing cakes and candies, consider these scenarios:
-
Sharing Resources: If you have 32 toys and want to divide them equally among 3 friends, understanding 32 divided by 3 will help you allocate the toys fairly. Each friend receives 10 toys, and you are left with 2 toys that you can keep or donate.
-
Crafting and Design: Architects utilize division when designing buildings, ensuring that spaces are allocated effectively. Dividing 32 square feet of floor space into 3 rooms might be necessary to optimize the layout and functionality of the design.
-
Cooking and Baking: Baking a cake, a recipe that calls for 32 ingredients, needs to be divided into thirds to create three batches for different occasions.
-
Data Analysis: Researchers may have 32 data points and need to divide them into 3 categories to analyze patterns and draw meaningful conclusions.

Image: otrabalhosocomecou.macae.rj.gov.br
Exploring Decimal Representation – A Closer Look
While the answer of 10 with a remainder of 2 provides a straightforward solution, it may not always be the most practical. We can delve deeper and express the result in decimal form. Here’s where the concept of “remainders as decimals” comes into play.
Dividing 32 by 3 results in a decimal representation of 10.666666… This infinite decimal, also known as a repeating decimal, reveals a deeper truth about the division process. It shows that while we can’t perfectly divide 32 into three equal parts with whole numbers, we can obtain a more precise representation by using decimal fractions.
The Importance of Remainders – Understanding the Leftovers
The remainder of 2 in our division problem holds significant meaning. It represents the “leftovers” that cannot be perfectly distributed among the three groups. This remainder is crucial for understanding the nuances of division and its implications in real-world applications. In the context of dividing a cake, the remainder would be the two bites that are leftover.
Leveraging the Power of Calculation: Practical Tips
-
Estimate and Round: In practical scenarios, it often suffices to approximate the result of division. We can round 10.666666… to the nearest whole number, resulting in 11. This method may be particularly useful when dealing with objects that cannot be split into fractions, like toys.
-
Embrace the Remainder: Instead of discarding the remainder, we can allocate it in a way that feels fair. For instance, when dividing a cake, the two leftover bites could be shared among the three individuals or saved for someone else.
-
Embrace Technology: Calculators and online resources offer powerful tools for performing complex calculations. They streamline the process of division, freeing up time for you to focus on the practical applications of the results.
32 Divided By 3
Conclusion: Unleashing the Power of Division
The humble act of dividing 32 by 3 encompasses a universe of mathematical concepts and practical applications. From understanding basic principles to exploring decimal representations and mastering real-world scenarios, this journey highlights the power and versatility of division. Whether you’re dividing a cake, allocating resources, or analyzing data, the insights gained from this exploration can empower you to make informed decisions and solve problems with clarity and efficiency. Embrace the world of division and unlock its potential to enrich your understanding of the world around you!





