Have you ever wondered how to measure the distance between two points on a coordinate plane? It’s a common question that arises in various fields, from geometry to physics. The distance formula is the key to finding that distance, and it’s a powerful tool that allows us to calculate the direct distance between any two points. In this blog post, we’ll dive deep into the world of the distance formula, and explore how it can be applied to real-world scenarios. Let’s unpack the distance formula and see how it’s used in math and beyond.
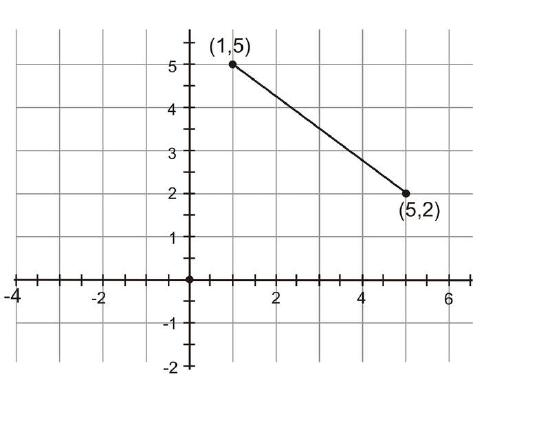
Image: k12.libretexts.org
Understanding the Distance Formula
The distance formula is a mathematical equation that helps us find the straight-line distance between two points in a two-dimensional coordinate plane. Essentially, it is derived from the Pythagorean Theorem, which states that in a right triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides. The distance formula leverages this theorem to calculate the distance between two points.
To grasp the concept, imagine you’re on a map. You want to know the direct distance between your current location and your destination. The distance formula allows you to calculate that distance by considering the horizontal and vertical differences between the two points.
Dissecting the Distance Formula
The Formula
The distance formula is represented as follows:
d = √((x₂ – x₁)² + (y₂ – y₁)² )
Where:
- d represents the distance between the two points.
- (x₁, y₁) represents the coordinates of the first point.
- (x₂, y₂) represents the coordinates of the second point.
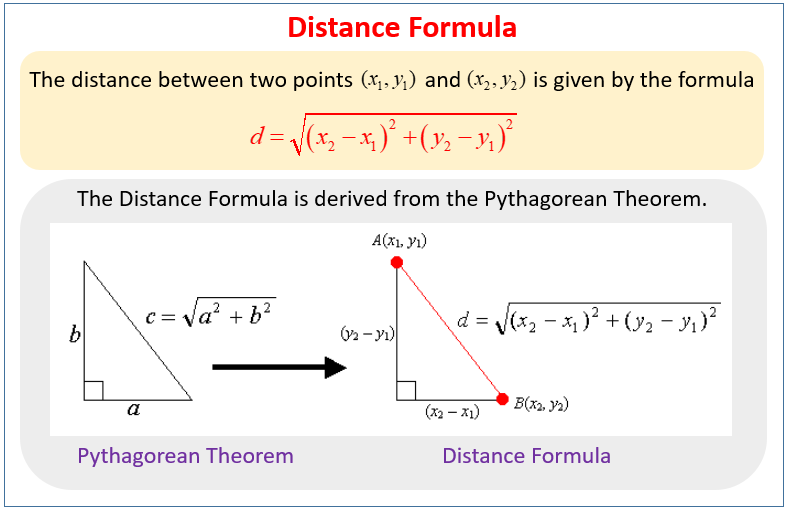
Image: lessondbjonathan.z21.web.core.windows.net
Breaking it Down
The formula essentially calculates the square root of the sum of the squared differences between the x-coordinates and the y-coordinates of the two points. Let’s break it down step-by-step:
- Find the difference in x-coordinates: Subtract the x-coordinate of the first point (x₁) from the x-coordinate of the second point (x₂).
- Square the difference: Square the result obtained in step 1.
- Find the difference in y-coordinates: Subtract the y-coordinate of the first point (y₁) from the y-coordinate of the second point (y₂).
- Square the difference: Square the result obtained in step 3.
- Add the squared differences: Add the value obtained from step 2 and the value obtained from step 4.
- Take the square root: Calculate the square root of the sum obtained in step 5. This will be the distance between the two points.
Applying the Distance Formula: Real-World Scenarios
The distance formula isn’t just a mathematical concept; it has numerous practical applications in various fields. Here are some examples:
- Navigation: GPS systems use the distance formula to calculate the shortest route between two points, incorporating road networks and traffic conditions.
- Construction: Architects and engineers utilize the distance formula to determine distances for building plans, bridge designs, and other structures.
- Robotics: Robotics engineers employ the distance formula to program robots to navigate obstacles and move through specific paths.
- Sports: Coaches and athletes use the distance formula to analyze player movements, calculate distances for throws and jumps, and optimize performance.
- Aviation: Air traffic control systems rely on the distance formula to track aircraft movements, maintain safe distances between planes, and manage air traffic flow.
Tips and Expert Advice
Here are some tips to help you master the distance formula:
- Visualize the Points: It’s often helpful to plot the points on a coordinate plane to understand their relative positions and the distance between them.
- Practice, Practice, Practice: Like any math concept, the best way to get comfortable with the distance formula is through practice. Solve numerous problems and gradually increase their complexity.
- Use Technology: There are online calculators and software that can help you solve distance formula problems. These tools can be useful for checking your answers and exploring different scenarios.
FAQ
Why is the distance formula important?
The distance formula is crucial because it provides a precise method for measuring distances between points in space. It’s a fundamental tool for numerous applications, from navigation and construction to robotics and sports.
How does the distance formula work?
The distance formula derives its foundation from the Pythagorean Theorem. It basically calculates the square root of the sum of the squared differences between the x-coordinates and the y-coordinates of two points.
Can the distance formula be used in three dimensions?
Yes, the distance formula can be extended to three dimensions. In this case, we would consider the differences between the x, y, and z coordinates of two points to compute the distance.
Are there any alternatives to the distance formula?
While the distance formula provides a standard approach, other methods can be used depending on the context. For instance, if you’re working with geographical coordinates, you might use the Haversine formula, which accounts for the Earth’s curvature.
1.2 4 Journal The Distance Formula
Conclusion
The distance formula is a fundamental mathematical tool with far-reaching applications in our daily lives. It allows us to calculate the distance between two points accurately, opening doors to numerous possibilities and advancements. Mastering the distance formula equips you with a versatile skill that can be utilized in various fields, enhancing your understanding of the world around you.
Are you interested in learning more about the distance formula or exploring its applications in other disciplines? Share your thoughts and questions in the comments section below!





